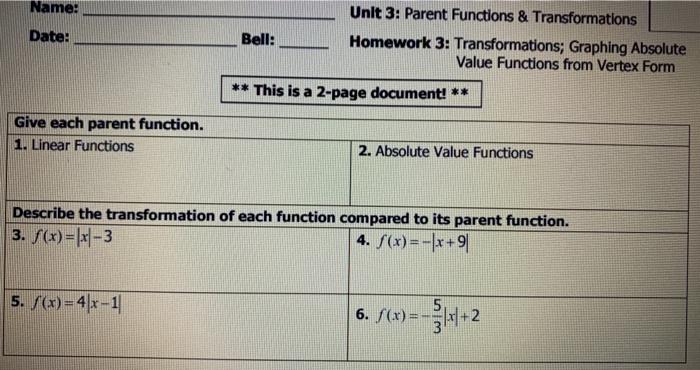
Unit 3 parent functions & transformations homework 1 piecewise functions – Unit 3: Parent Functions and Transformations Homework 1: Piecewise Functions delves into the fascinating world of piecewise functions, exploring their definition, types, graphing techniques, applications, transformations, and comparisons. This comprehensive guide provides a thorough understanding of these versatile functions and their significance in real-world scenarios.
Piecewise functions are a unique class of functions defined by different rules over different intervals of the domain. They offer a powerful tool for modeling complex relationships and phenomena, making them indispensable in various fields.
Piecewise Functions
Piecewise functions are functions that are defined differently over different intervals of their domain. They are commonly used to model situations where the behavior of the function changes abruptly at certain points.
Types of Piecewise Functions, Unit 3 parent functions & transformations homework 1 piecewise functions
- Constant functions:These functions have the same value for all values in their domain.
- Linear functions:These functions are defined by a straight line over each interval of their domain.
- Absolute value functions:These functions have a V-shaped graph over each interval of their domain.
- Step functions:These functions have a constant value over each interval of their domain, but the value changes abruptly at the endpoints of each interval.
Graphing Piecewise Functions
To graph a piecewise function, follow these steps:
- Identify the different intervals of the function’s domain.
- Determine the function rule for each interval.
- Graph each piece of the function over its corresponding interval.
- Connect the pieces of the graph at the endpoints of each interval.
Applications of Piecewise Functions
Piecewise functions are used in a variety of applications, including:
- Modeling piecewise linear functions
- Defining piecewise constant functions
- Creating piecewise absolute value functions
- Modeling step functions
Transformations of Piecewise Functions
Piecewise functions can be transformed in the same way as other functions. The following transformations can be applied to piecewise functions:
- Translations
- Reflections
- Stretching and shrinking
Comparing Piecewise Functions
| Type | Definition | Graph | Applications |
|---|---|---|---|
| Constant | f(x) = c | Horizontal line | Modeling constant values |
| Linear | f(x) = mx + b | Straight line | Modeling linear relationships |
| Absolute value | f(x) = |x| | V-shaped graph | Modeling absolute values |
| Step | f(x) = a if x < c, b if x ≥ c | Horizontal lines with abrupt changes | Modeling discontinuous functions |
Commonly Asked Questions: Unit 3 Parent Functions & Transformations Homework 1 Piecewise Functions
What are piecewise functions?
Piecewise functions are functions defined by different rules over different intervals of the domain.
What are the different types of piecewise functions?
Common types of piecewise functions include constant functions, linear functions, absolute value functions, and step functions.
How do you graph piecewise functions?
To graph piecewise functions, identify the different intervals and apply the corresponding rules to each interval.
What are some real-world applications of piecewise functions?
Piecewise functions are used in various fields, such as economics (modeling piecewise linear demand curves), physics (describing piecewise constant velocity), and computer science (defining piecewise continuous functions).